Maurits Escher’s impossible reality
Maurits Escher is a "mathemagician" who has created realistic yet physically impossible constructions that combine art and mathematics. His unique and original style is based on the manipulation of shapes, spaces and perspectives, exploring themes such as impossible constructions, exploring infinity, tessellations and metamorphoses.
From landscapes to architecture, a graphic journey
Maurits Cornelis Escher was a Dutch draughtsman, book illustrator and tapestry designer, best known as a printmaker. Born on June 17, 1898, he died in 1972 in the Netherlands, leaving behind more than 400 prints and 2,000 drawings. Until the age of 13, he apprenticed as a carpenter, which gave him notions of 3D spatial construction. Initially inspired by architecture, he went to a school specializing in architecture and art in Haarlem. He failed his exams due to health problems, before finally choosing decorative arts under the guidance of Samuel Jessurun de Mesquita, an artist who introduced him to woodcutting and with whom he remained close until this friend and his family were decimated by the Nazis in 1944.
Below, engravings by Mesquita, followed by works from Escher. The two artists' work is characterized by clear lines, simplicity in contrasting solid forms, and geometry.
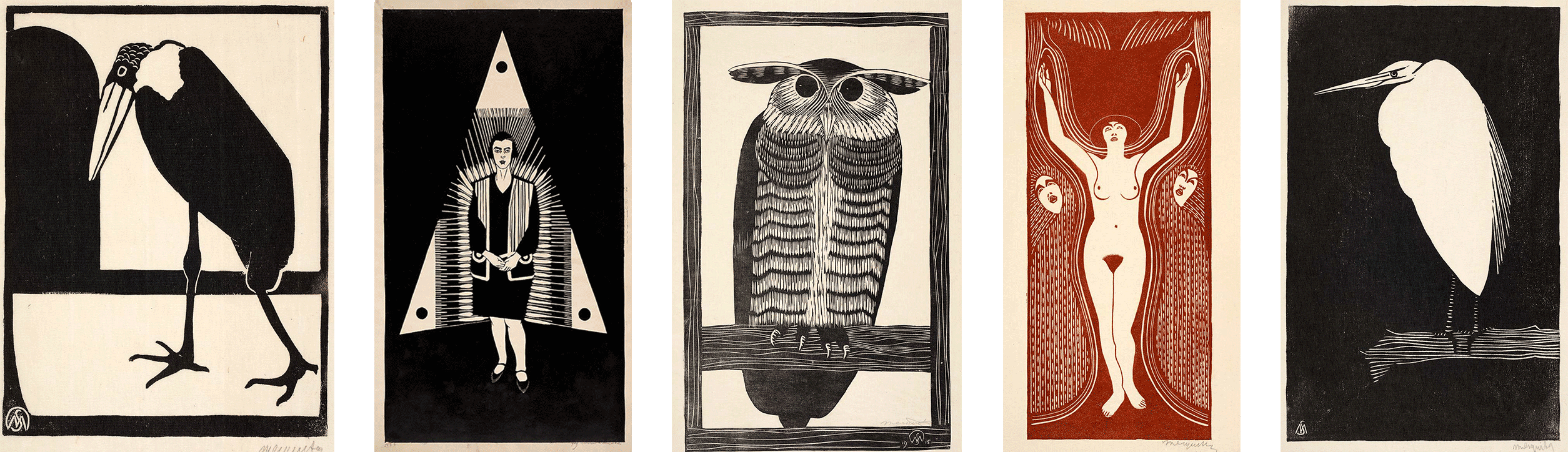 Engravings by Samuel Jessurun de Mesquita: Marabout, 1909 - Woman, 1929 - Owl, 1915 - Ecstasy, 1922 - Heron, 1928
Engravings by Samuel Jessurun de Mesquita: Marabout, 1909 - Woman, 1929 - Owl, 1915 - Ecstasy, 1922 - Heron, 1928
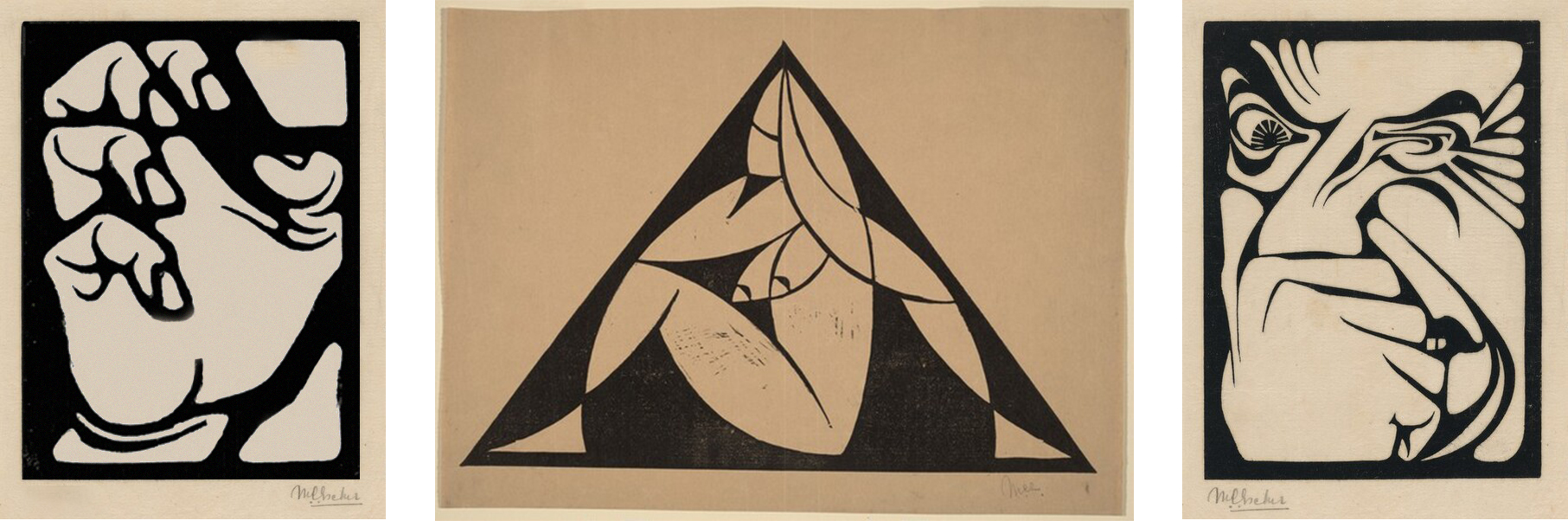 Prints by M.C. Escher, 1921: Whore's Superstition - Woman Sitting Naked - Perfume
Prints by M.C. Escher, 1921: Whore's Superstition - Woman Sitting Naked - Perfume
Escher also drew inspiration from Giovanni Battista Piranesi, an 18th-century Italian engraver famous for his fantastic, dizzying views of Rome and his imaginary prisons.
The Italian countryside, with its dense cities, dramatic coastlines and winding roads, was M.C. Escher's primary inspiration for drawings. With these landscapes, he explored the technique of "scratch drawing", covering his sheet with lithographic ink (a fatty ink), which he then scratched with a tip. From his studio in Rome, where he lived and engraved, he drew inspiration from the details of buildings, sometimes composing astonishing, simultaneous perspectives in two opposite directions.
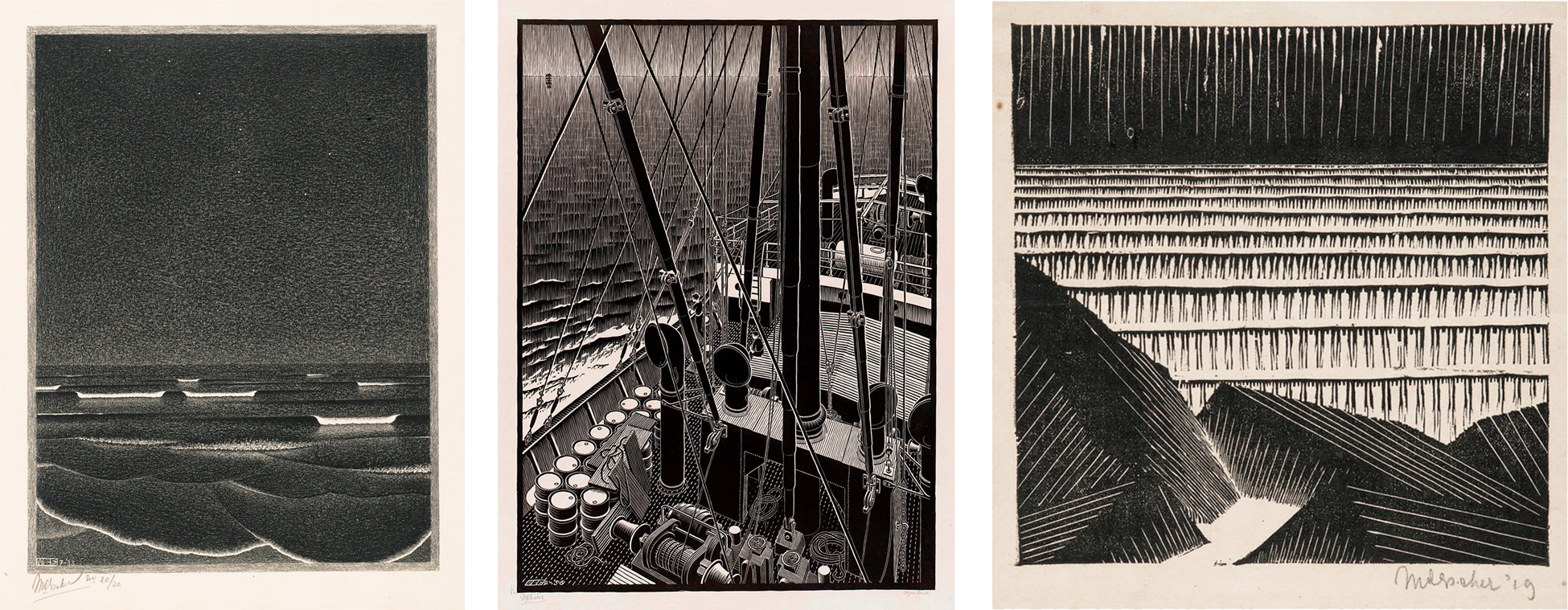
In 1922 and again in 1936, Maurits Escher visited Spain, whose architecture had a profound influence on his work. Escher was also inspired by Flemish primitive painters, optical games (we'll talk about these two inspirations below), medieval and fantasy bestiary (he drew many dragons and mythological beasts), and surrealism, of which he was a contemporary. All through his there is a certain fascination with infinity, whether in his tessellations or in his mathematical drawings.


The beauty of Islamic art monuments, particularly the repetitive and geometric patterns of the tiles in the Alhambra in Granada, a 14th-century Moorish palace, was a revelation. The recesses and interlocking elements of bas-reliefs, battlements, and tiles inspired him to create abstract and whimsical forms, playing with negative/positive space to the extent that tessellation became a true obsession. In Andalusia, he also studied the Mezquita Mosque in Cordoba, from which he made several observation sketches.


With his wife and children, Escher lived in Italy (which they left with the rise of fascism), Switzerland, then Belgium before returning to the Netherlands in 1941.
A Master of Printmaking
Escher employed various techniques in his career, such as wood engraving in the early years, mezzotint from 1930 onward (a type of engraving with a very subtle grain that avoids using hatching or small dots for half-tones, creating rich and deep tones), lithography (based on the chemical principle of the repulsion of grease and water, where one draws on an absorbent limestone with a greasy pencil or brush, then moistens the stone and inks it, allowing the drawn parts to retain ink for printing), as well as linoleum engraving (in which the soft support is carved with tools before inking what remains on the surface for printing). Escher also illustrated books, tapestries, stamps, and murals.
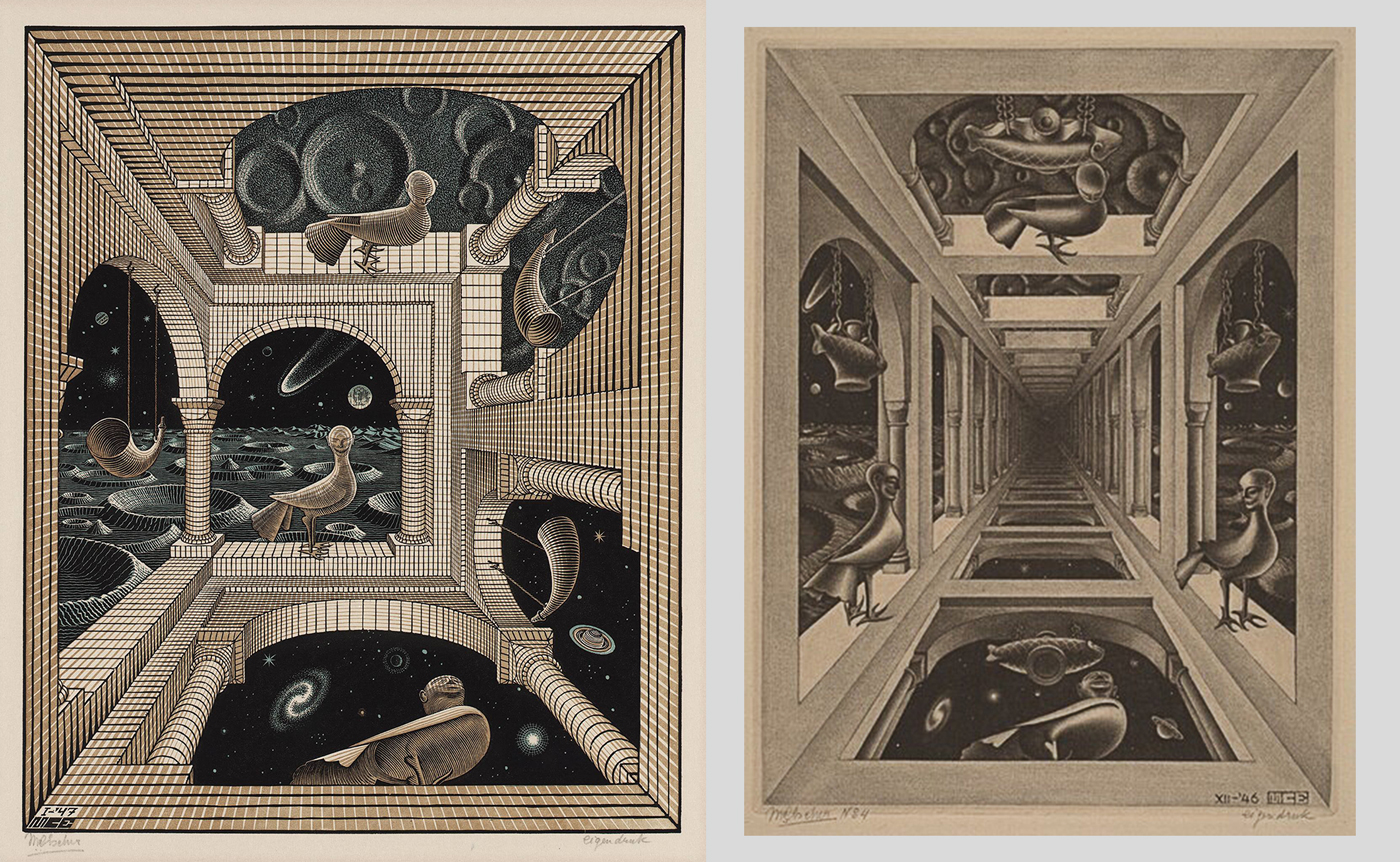 Another World, M.C. Escher. On the left, a lithograph from 1947, reproducing the impossible architecture of the mezzotint on the right, 1946
Another World, M.C. Escher. On the left, a lithograph from 1947, reproducing the impossible architecture of the mezzotint on the right, 1946
An Obsession with Geometric Tessellations
In the 1920s, following his trip to the Alhambra while still a student, he developed a theory: the "regular division of the plane." He demonstrated that by using repetitive and geometric patterns, one could cut space without leaving gaps between each shape, creating periodic tessellations. This technique is also used in the tiling of decorative patterns and in crystallography to represent the physical forms of crystal atoms. His brother, Berend George, a pioneer in experimental geology, introduced him to crystallography. In 1922, Escher created his first motif composed of eight heads (shown below), which he printed separately in nested squares:
 M.C. Escher, eight heads, 1922, wood engraving
M.C. Escher, eight heads, 1922, wood engraving
In crystallography, a crystal's structure revolves around a repeated unit (in Escher's case, a motif) in all directions, with symmetry properties such as center symmetry, inverse or direct rotation axes, or mirrors. This allows the infinite and gapless repetition of the motif, represented in 3D by grids of cubes (as shown below) or in 2D by periodic tessellations.
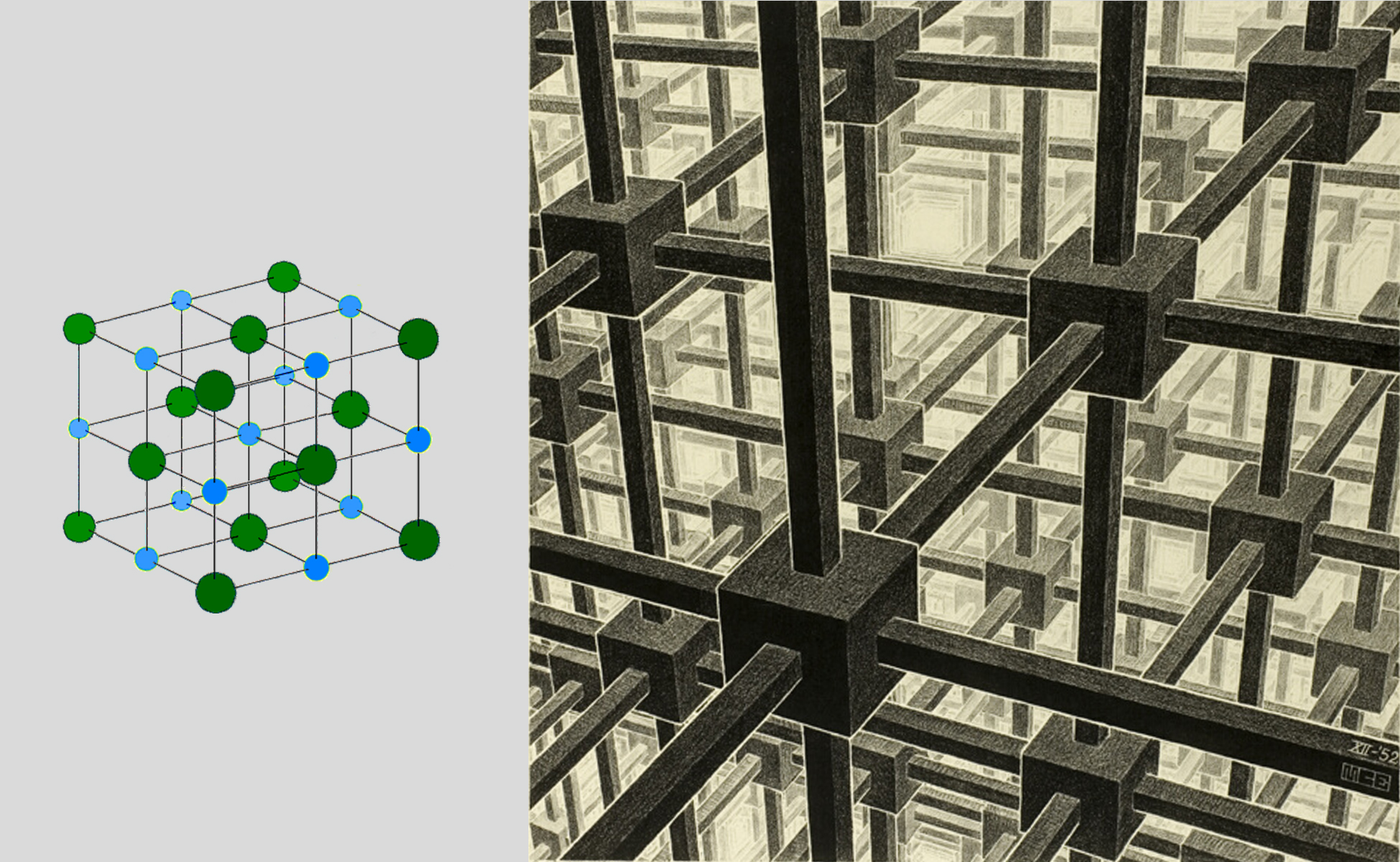 Crystallography of a fossilized sea salt atom — Cubic division of space, 1952
Crystallography of a fossilized sea salt atom — Cubic division of space, 1952
Escher explained his theory of regular plane division as follows: "A plane, which should be considered as unlimited from all sides, can be filled or divided into similar geometric figures that adjoin each other on all sides without leaving empty spaces. This can be continued to infinity according to a limited number of systems." He played with various shapes that he nested or evolved.
 M.C. Escher, Flying Fish, 1954 — Bird-Fish, 1938
M.C. Escher, Flying Fish, 1954 — Bird-Fish, 1938
During the same period, after his first trip to Andalusia, Escher began a series of prints called "metamorphoses," in which he transformed one form, animal, or character into another; for example, a bird into a donkey. In the visual below, there is a series starting from the fortified city to the Chinese doll (1937), followed by another metamorphosis from 1939-1940, cut into 4 sections here to see the details, from checkerboard to lizards, hive, bees, fish, birds, checkerboard, and city roofs before ending in a chessboard.

This pursuit of form mutation associated with gapless tessellations led him to develop mathematical systems of patterns that evolve infinitely.
 M.C. Escher, Horses and Birds, 1949 — Verbum, 1942
M.C. Escher, Horses and Birds, 1949 — Verbum, 1942
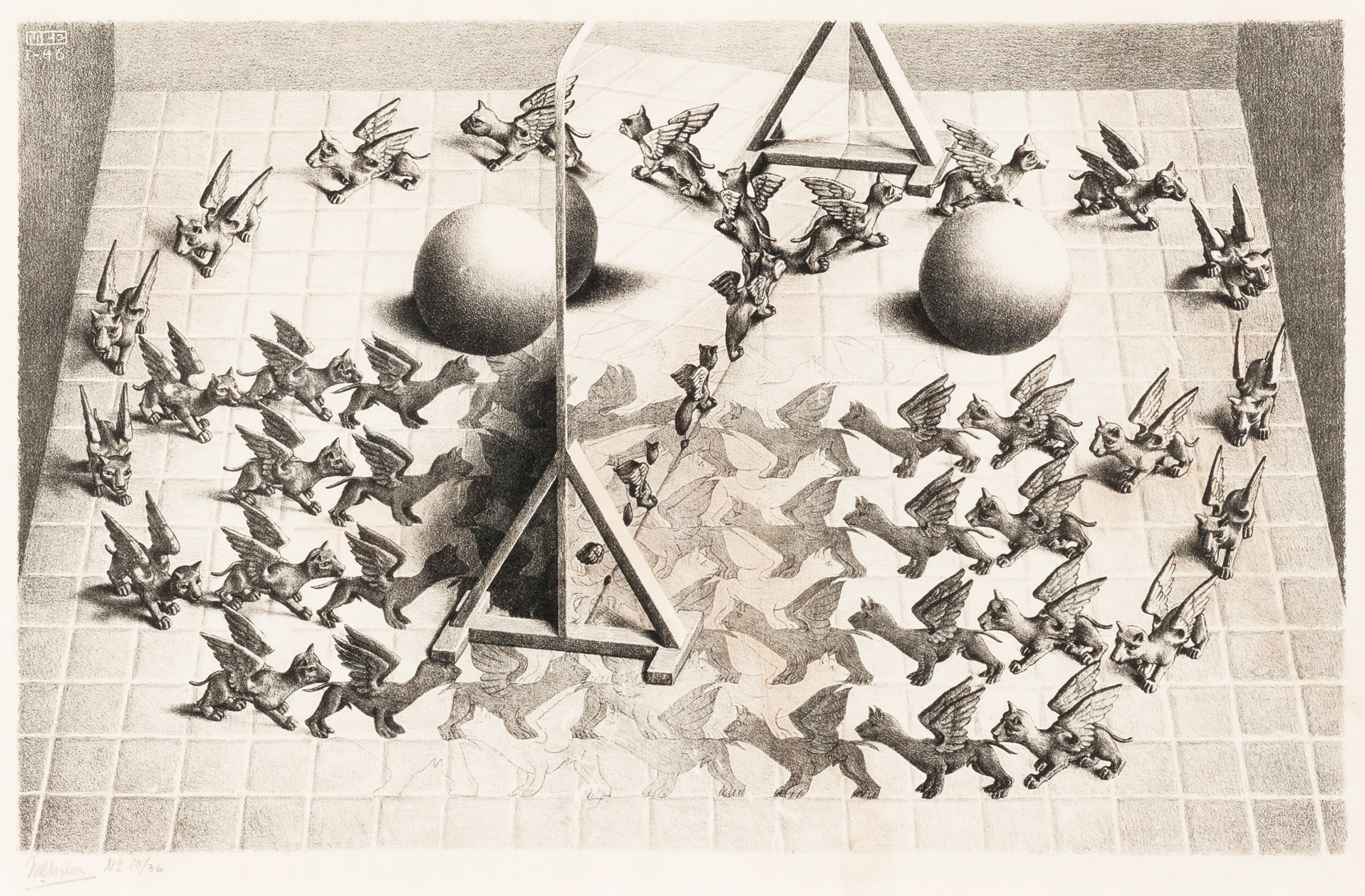 M.C. Escher, Magic Mirror, 1946
M.C. Escher, Magic Mirror, 1946
 M.C. Escher, Knights, 1957 - 1946
M.C. Escher, Knights, 1957 - 1946
Leaving Italy in 1935, he abandoned the study of landscapes to create what he called "mental imagery," the art of creating visual representations that defy the laws of physics, logic, and perspective...
Trompe-l'oeil and Mathematical Magic
A magician fascinated by mathematics, which he considered the source of beauty and harmony, Escher studied geometry, topology, logic, fractals, complex numbers, and dimensions. He used mathematics to create surprising visual effects, such as paradoxes, illusions, and infinite shapes. Georges Perec wrote about trompe-l'oeil in The Dazzled Eye: "For a moment, we were made to doubt our senses, and in this brief and ephemeral mystification, something magical, marvelous, a delightfully Borgesian astonishment is revealed..."
There are several "impossible objects" or "paradoxes" among his sources of inspiration, such as the Penrose triangle and staircase, the Möbius strip, and the Necker cube, four 2D shapes that give the illusion of 3D thanks to optical games, but which cannot exist in volume in the real world. His inspiration lead him to collaborate with mathematicians and scientists, who also used his work to illustrate their concepts, and offered him ideas and models.

As Gilles Methel writes in "Un art magique?" (Entrelacs): "It is not the representation of space that is in question but the way of inhabiting it by defying the laws of gravity." Maurits Escher used mathematics to philosophize about the absurdity of passing time and space, often represented in an infinite loop that borders on the absurd. Waterfalls that rise, staircases that follow one another in endless circles, animals that change shape and reincarnate... his imagination transcends the limits of the brain in mind-bending illusions.
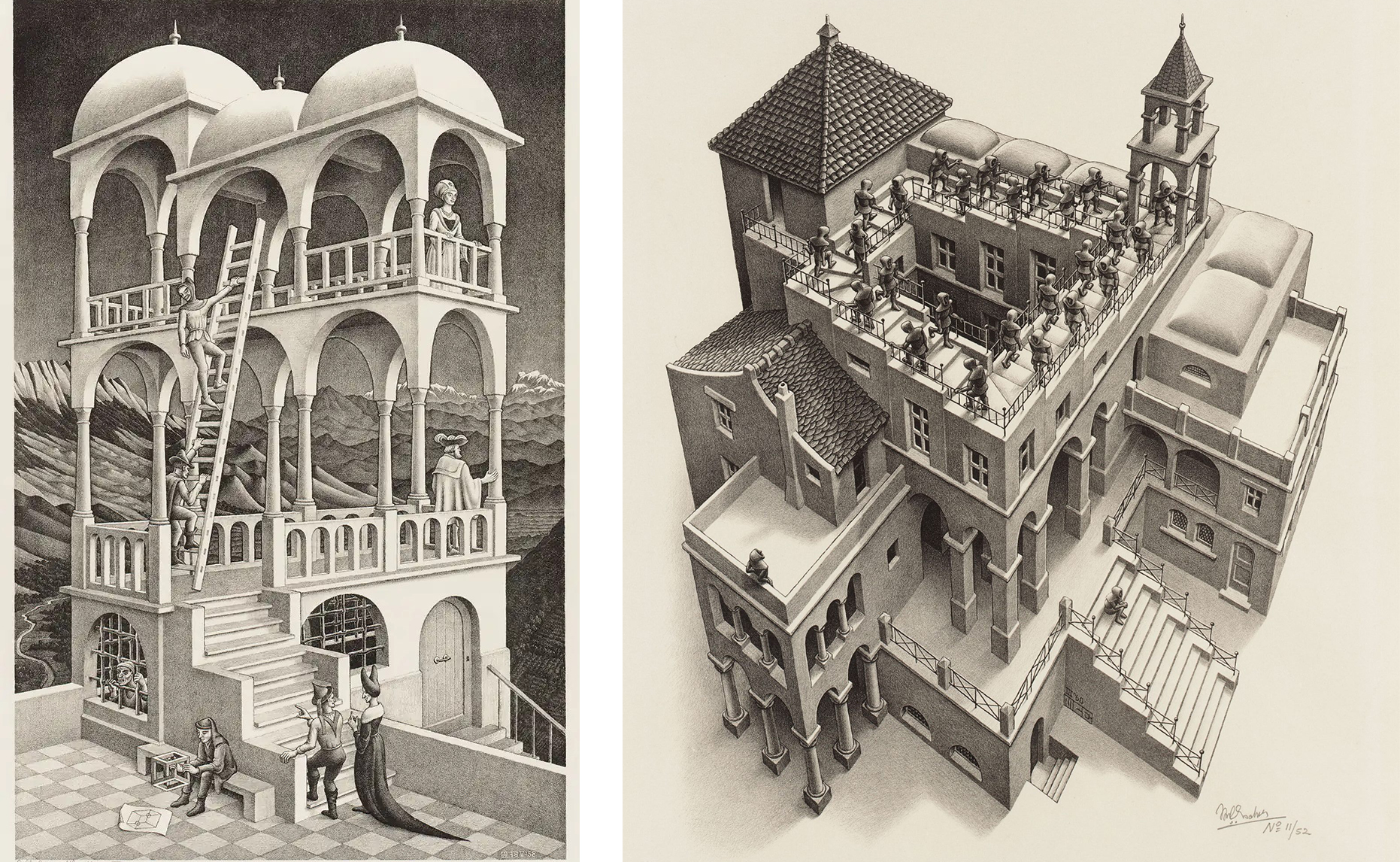 M.C. Escher, Belvedere, 1958 — Ascending and Descending, 1960
M.C. Escher, Belvedere, 1958 — Ascending and Descending, 1960
Escher also drew inspiration from Poincaré's hyperbolic geometry by endlessly repeating patterns in spheres, similar to stereographic projections used in crystallography. The term fractals, explaining this principle of repeating a pattern at variable scales, was coined two years after Escher's death.

Spheres and Reflections
Escher developed an impressive ability to draw spheres and, notably, reflections, distorting perspective into curves. His methods were so precise and perfect that it seemed as if he were using software, but everything was done by hand. He drew inspiration from the great Flemish masters of the 15th century (being Flemish himself), such as Jan Van Eyck, Quentin Metsys, or Petrus Christus, who painted numerous pictures with convex mirrors. He was also influenced by a self-portrait of the Italian painter Parmigianino from the 16th century. Achieving such a result was a testament to his great mastery of curves, especially since he engraved his motifs, making the work even more meticulous than drawing.
 Quinten Metsys, The Moneylender and His Wife (detail), 1514 — Jan van Eyck, Portrait of the Arnolfini Couple (detail), 1434 — Parmigianino, Self-Portrait in a Convex Mirror, 1524 — Christus, A Goldsmith in His Shop, Possibly St. Eligius (detail), 1449
Quinten Metsys, The Moneylender and His Wife (detail), 1514 — Jan van Eyck, Portrait of the Arnolfini Couple (detail), 1434 — Parmigianino, Self-Portrait in a Convex Mirror, 1524 — Christus, A Goldsmith in His Shop, Possibly St. Eligius (detail), 1449
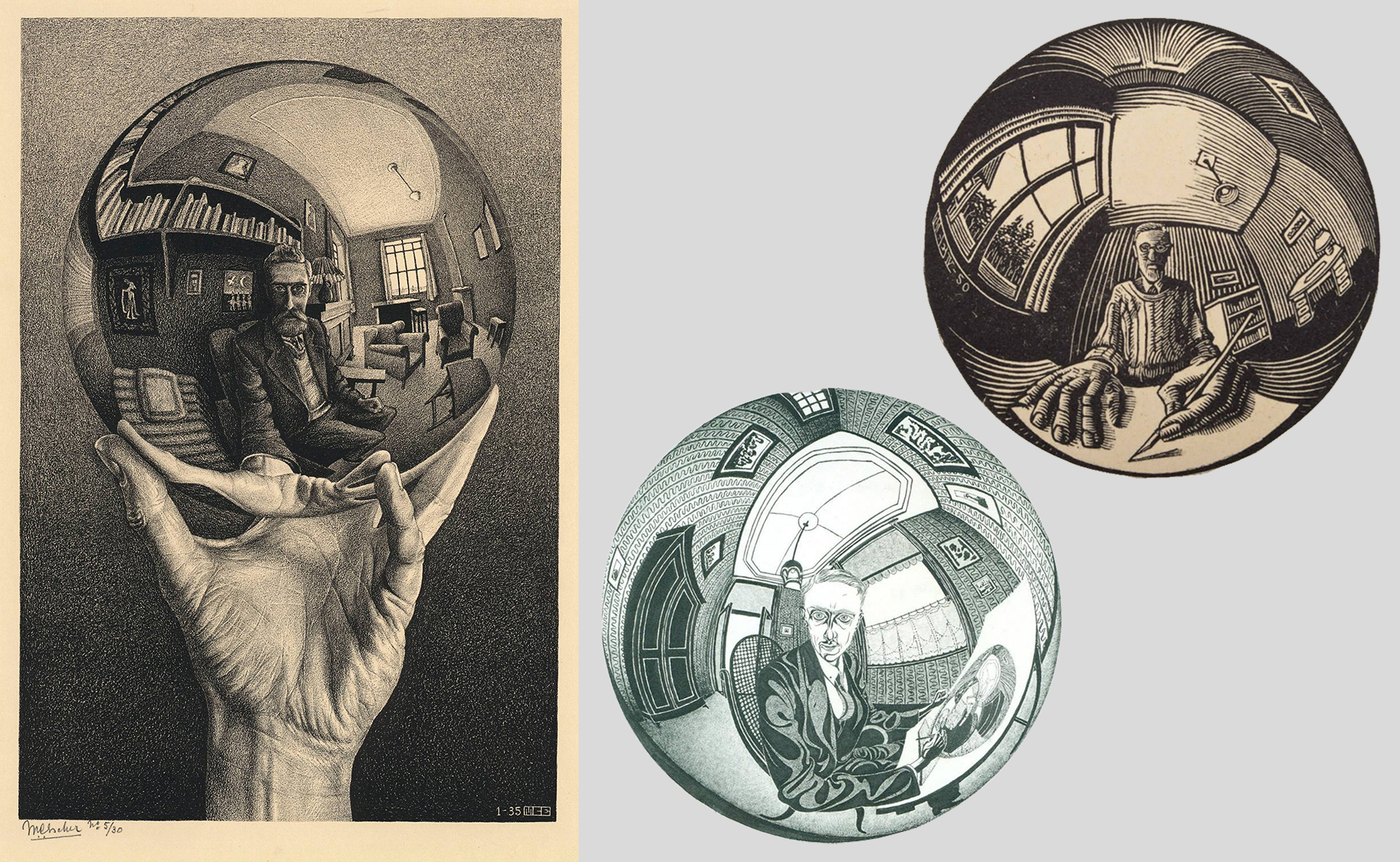 M.C. Escher, Hand with Reflecting Sphere, 1935 — Self-portraits
M.C. Escher, Hand with Reflecting Sphere, 1935 — Self-portraits

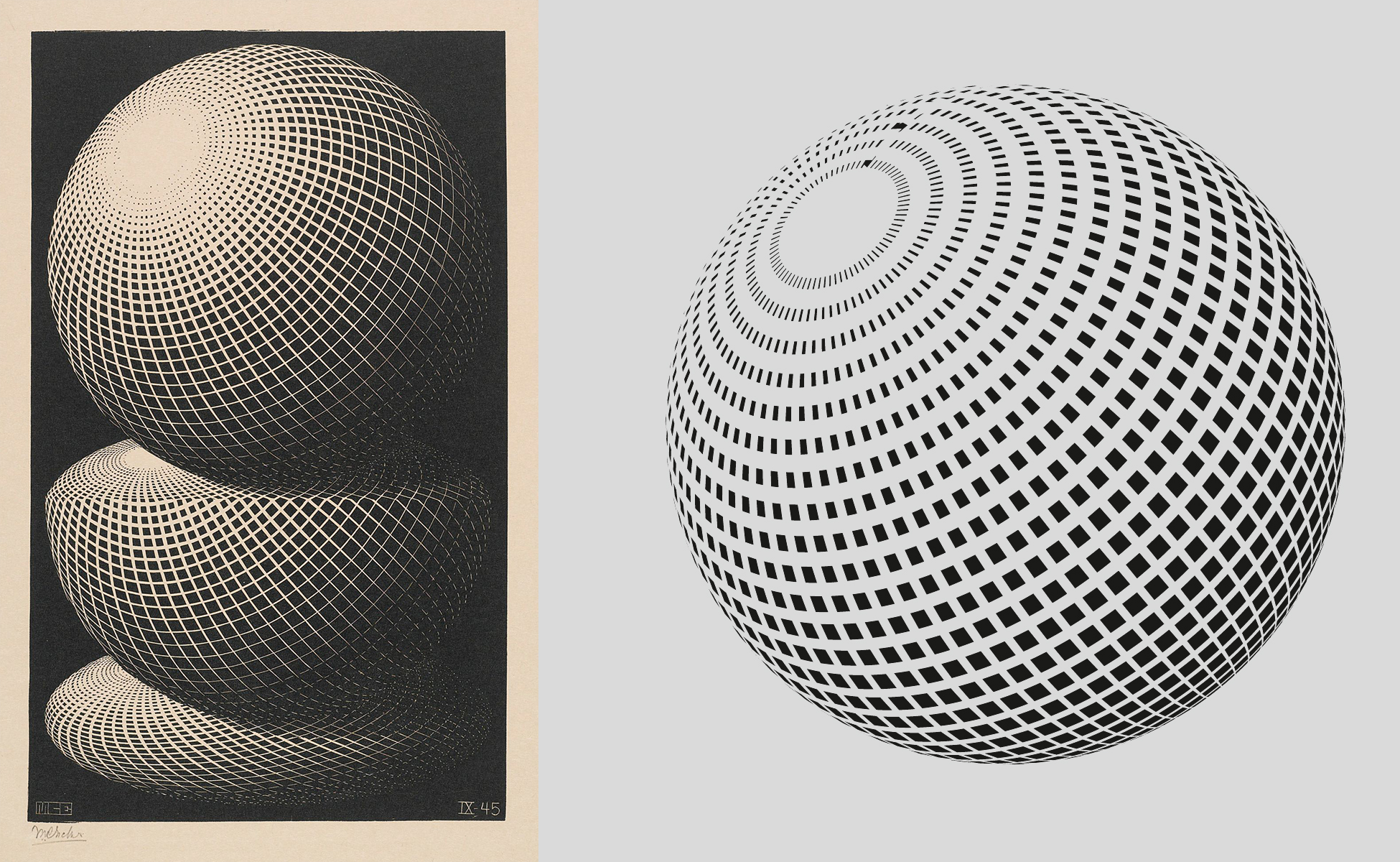
M.C. Escher, Three Spheres, 1946
Today, one can create a nearly similar rendition of the work "Three Spheres" (1945) in Illustrator in a few minutes (or hours)... Create an arc, select the 3D "revolve" option, and check "straight edge." Then, create a pattern of white triangle-shaped lines on a black rectangle background and apply it in "overlay" to the sphere. It's relatively straightforward in theory, but creating the right pattern may take some time, just give it a try. Ours is not perfect, by the way. The 3D options also allow you to add any texture to a sphere, in a few clicks.
Curved and Infinite Perspective
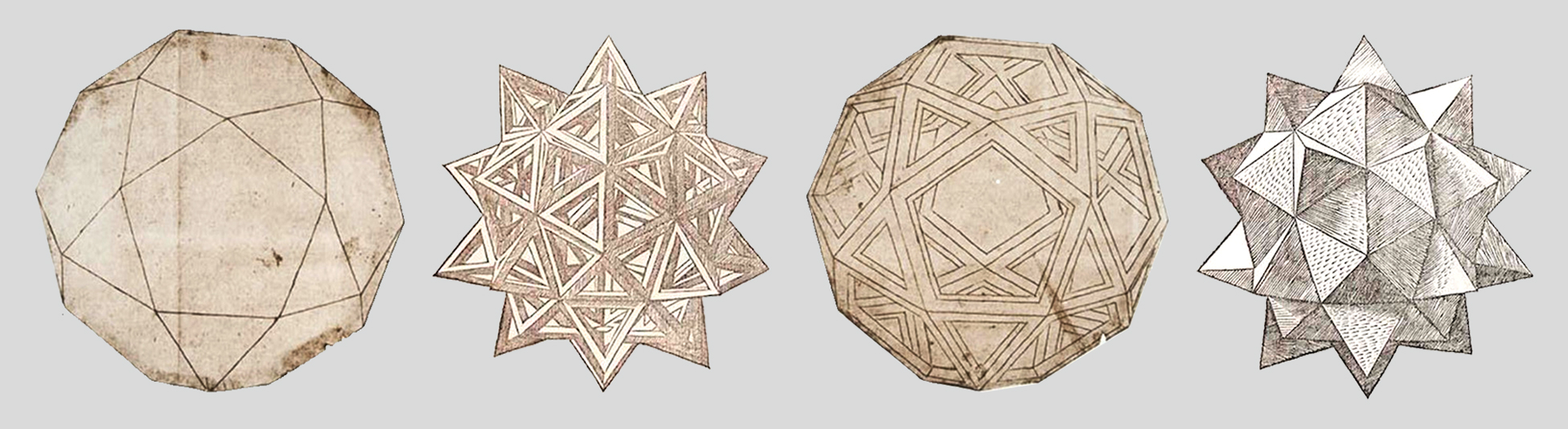
Nicholas of Cusa, in The Painting or Vision of God in 1453, wrote that "The angle described by your eye, my God, is not limited in space but is infinite; it is the circle, even more: the infinite sphere. For your gaze is the eye of sphericity and infinite perfection." Without considering himself to be God, Escher was well aware that mathematics and the divine have often been linked, especially in the 15th century, when the Christian Creator was considered to be "the world's great watchmaker", and mathematics was his language of expression. Pacioli and Da Vinci's joint work, "De Divina Proportione,", in which Da Vinci illustrates the "divine" proportions of mathematical figures or even Roman typefaces, was a great source of inspiration for Escher (illustrations below, with Da Vinci's illustrations in line 1, and Escher's below), who used the geometric forms in his very handmade art.
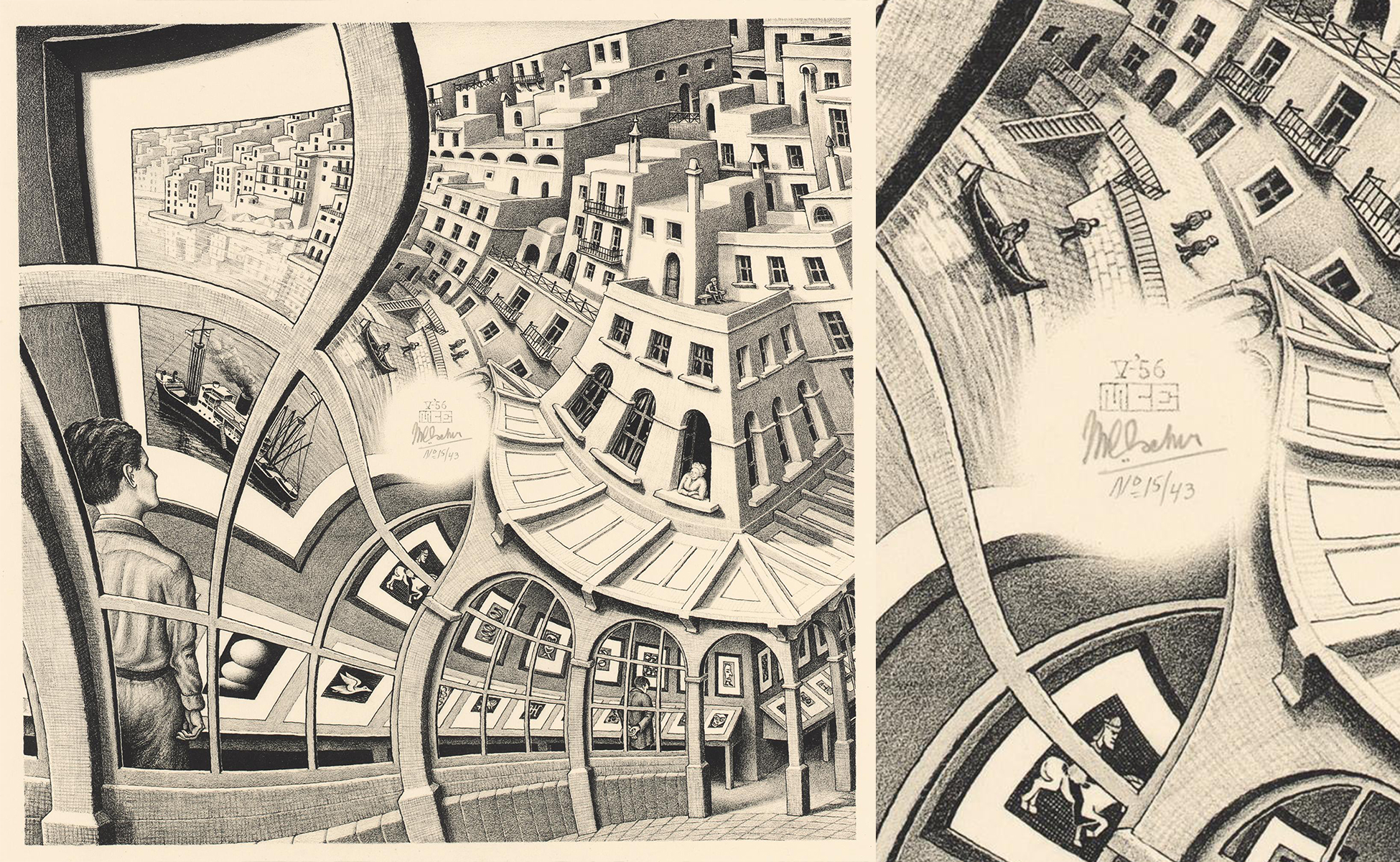
With spheres, he tackled straight-line perspective, which he believed was incorrect because it does not consider retinal perspective, which, according to him, is curved. He called this finding "cylindrical perspective" and demonstrated it in an unfinished work, "Exhibition of Prints," in 1956, leaving the central point blank (with his signature in the center). The work was completed in 2004 by a team of mathematicians from the University of Leiden and Dutch artist Jacqueline Hoftra, who observed a combination of mathematical functions following the angles of Escher's grid, creating an infinite spiral in the painting. This Droste effect, a form of recursion, comes from Droste cocoa's advertisement, in which a nun holds a tray with a box of cocoa powder on it, with the same image appearing on the box, creating an infinite visual loop. Faced with the perplexity he often generated in his admirers, Escher liked to respond, "All this is nothing compared to what I see in my head!"
Escher graphic designer
It's less well known, but M.C. Escher also created several logos and illustrations for books, as a graphic designer. He also designed a few of stationery gifts (top right for the Zingone brand and left for de Bijenkorf, in 1933), and greeting cards for friends of his.
Shown below in red is an extremely detailed logo for a Chinese and Indian restaurant (1944), one of his first linocuts for an ex-libris depicting a knight in green and black (1918), another with two storks (1917), a third woodcut ex-libris from 1946, top left, and three posters, bottom right, for exhibition invitations and a chess club (1931 and 1936). Click on the image for a larger view.
 Greeting cards for Eugène and Willy Strens, earth element in 1953, air element in 1954, Escher, wood engravings.
Greeting cards for Eugène and Willy Strens, earth element in 1953, air element in 1954, Escher, wood engravings.
 Greeting cards for Eugène and Willy Strens, fire element in 1956, and water element in 1956, Escher, wood engravings.
Greeting cards for Eugène and Willy Strens, fire element in 1956, and water element in 1956, Escher, wood engravings.
Escher was long neglected by the art world, which regarded him as a mere extra-plastic illustrator or a popular artist. It was only towards the end of his life that he began to receive international recognition and critical appreciation. At the age of 70, his works began to be exhibited in numerous museums and galleries, and they were reproduced in many books and articles. Today, he is renowned and recognized in many countries.
Escher's Influence in Cinema, Games, and Logos
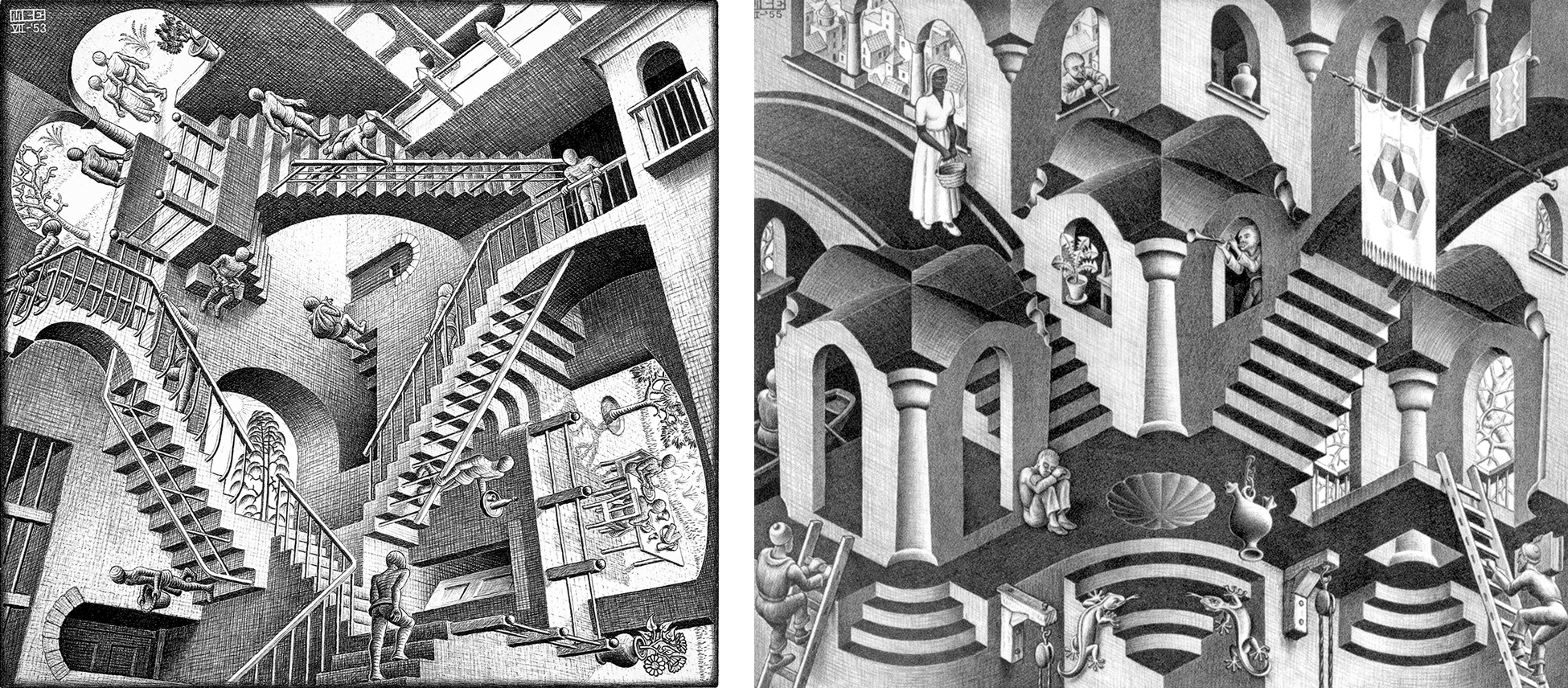
Escher's works have also influenced popular culture, including cinema, music, literature, video games, and logos. The lithographs most often referenced are "Relativity" (1953) with its impossible stairs and "Convex and Concave" below (1955), in which the stairs lead both up and down, creating a perfect illusion:
This principle of impossible floors can be found, for example, in Jim Henson's strange 1986 film Labyrinth, starring David Bowie, which makes a mockery of perspective:

...but also in Shawn Levy's Night at the Museum 3: Secret of the Tomb (2014), in which the main character dives into Relativity. The spiral staircase scene in Harry Potter and the Philosopher's Stone is also a reference.

Christopher Nolan, mentioned in our article on ambigrams, is also a big fan of Escher's engravings. He adapts Escher's works, such as "Gravity," "Relativity," "Cubic Division of Space," or "House of Stairs," in "Inception" (2010) with the city folding onto itself and in "Interstellar" (2014) with the Cooper Station or the tesseract scene reminiscent of Escher's 3D crystallographic structures.
 The Tesseract, Interstellar, a scene reminiscent of the 3D structure of "Cubic Division of Space" by Escher
The Tesseract, Interstellar, a scene reminiscent of the 3D structure of "Cubic Division of Space" by Escher
Nolan mentioned being inspired by "how things connect or blur the boundaries between art and science, and art and mathematics" in Escher's works.

Escher's influence is also evident in "The Lord of the Rings," with the Mines of Moria or the departure of the elves (hello fans) reminiscent of the Crypt Procession of 1927, and even Bilbo's evening fireworks, inspired by the 1933 engraving.
More recently, Escher's influence has surfaced in the "Squid Game" series, where the set design incorporates the perspectives of impossible staircases. In the realm of games, "Monument Valley," discussed in our article about graphic mobile games, is based on impossible perspectives with winding stairs that open up new paths.
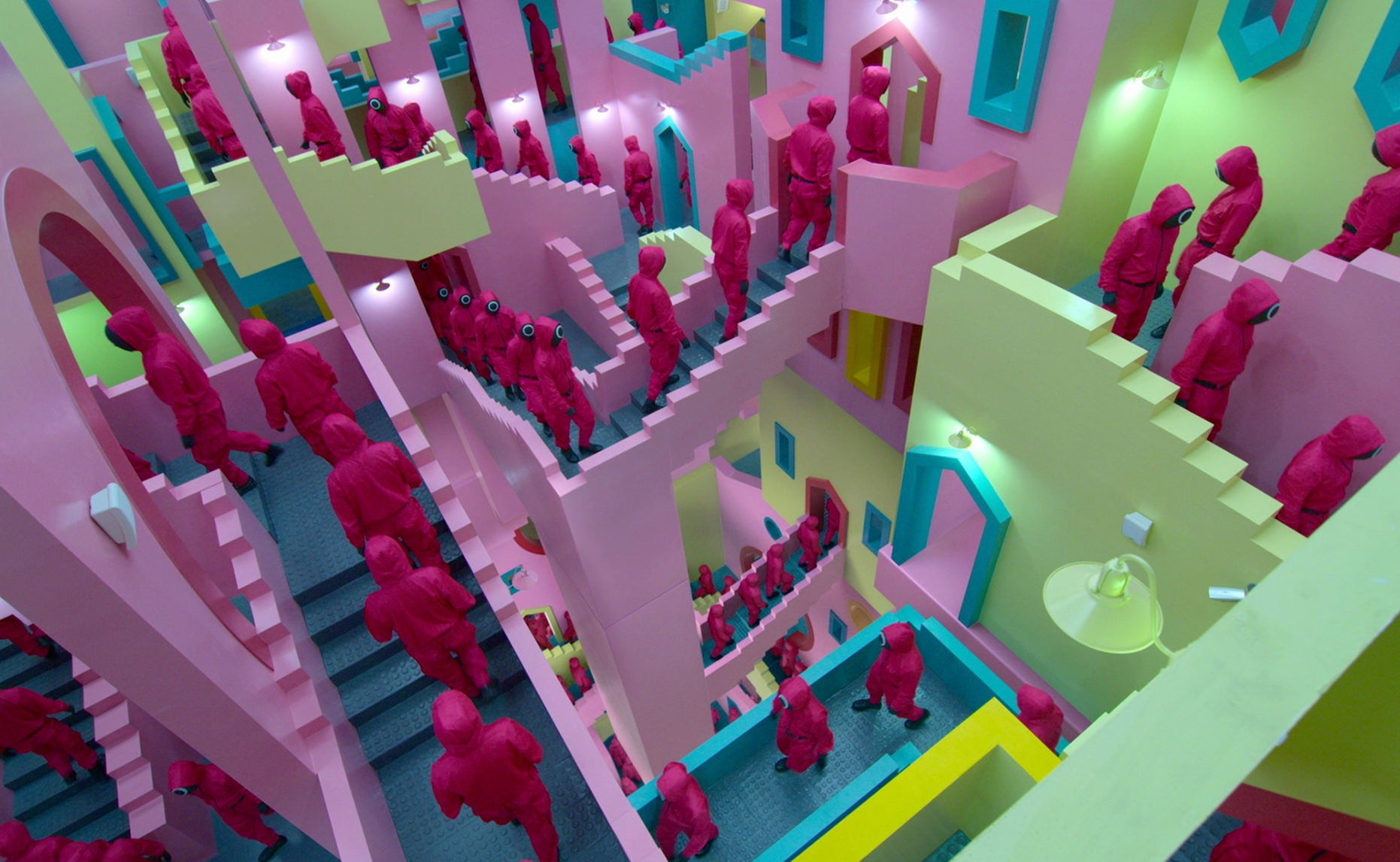

An enthusiast has even created a Lego rendition of Escher's work. There are likely hundreds more references, so feel free to share them in the comments.

Logos and inspiration
On the logo side, one might enjoy imagining a connection between the peel of Orangina and Escher's Peel, but the former debuted in 1953, two years before the artwork. At the time of creating the orange peel logo, French regulations prohibited the representation of fruits on products containing less than 25% juice. The graphic designer, Villemot, got around this by depicting the fruit's peel!

Similarly, the Woolmark logo by Grignani (1964) seems to echo Escher's Knot (1965), but the two apparently share no direct link, except for an affinity for lines and geometric forms!

Escher is regarded as one of the greatest graphic artists of the 20th century, mastering illusion and geometry long before the advent of computer-generated images. His boundless patience gave rise to fascinating and enigmatic works that defy logic and imagination, inviting reflection and wonder. "I cannot help admiring the laws of nature, which reveal themselves in the smallest things," he once said.
More sources :
Escher in heit paleis
http://sweetrandomscience.blogspot.com/2012/11/escher-et-les-sciences-lobsession-de.html
https://www.illustrationhistory.org/essays/the-hidden-emotions-in-m.c-eschers-artwork
https://journals.openedition.org/entrelacs/2015
Share this post:











 San Serriffe typographic Island
San Serriffe typographic Island Design, creativity and oblique strategies!
Design, creativity and oblique strategies! Tote bag, a new social totem?
Tote bag, a new social totem? Sister Corita Kent, the Pop Art nun
Sister Corita Kent, the Pop Art nun Donald Trump, the martyr who makes history
Donald Trump, the martyr who makes history Museum-Memorial of Terrorism – Visual identity
Museum-Memorial of Terrorism – Visual identity Ilex, paysage+urbanisme
Ilex, paysage+urbanisme Groupe iliad – Visual identity
Groupe iliad – Visual identity Répar’acteurs –Visual identity
Répar’acteurs –Visual identity Science Forum communication
Science Forum communication CNFPT Visual Identity
CNFPT Visual Identity Typorama #05 : Times after time
Typorama #05 : Times after time Natalie Portman, serial killer for Dior Rouge
Natalie Portman, serial killer for Dior Rouge Ski trail map designer: such a cool job!
Ski trail map designer: such a cool job! We ❤️ Milton Glaser !
We ❤️ Milton Glaser !

Leave a Reply